Find the Slope From Two Points on the ISEE
Middle and Upper Level
ISEE Question: What is the slope of the line that includes the points (0, 2) and (2, -5)?
A) -4
B) -7/2
C) -1
D) 3
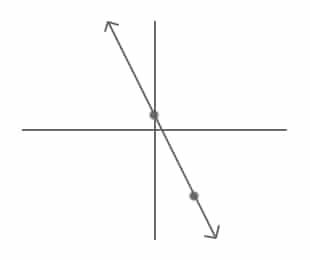
Quick Strategy: Make a rough sketch of the two points on the coordinate plane to see the direction and general steepness of the line. In the sketch below, I can tell that the slope of the line is negative and steeper than -1, so I can eliminate C and D. Don’t take time to do an exact drawing.
Exact Solution: Use the slope formula.
The formula for slope is 
This can also be defined as 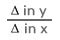
Another way to write this formula is 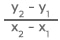
STEP 1: In order to not get mixed up, label each number in your set of ordered pairs:
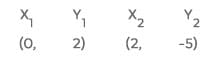
Helpful Tip: it doesn’t matter which pair is “1” and which pair is “2,” as long as you’re consistent during your computation. If I reverse the order for the ordered pair above, I get
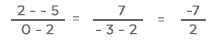
STEP 2: Plug your numbers into the slope formula, and then simplify.
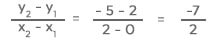
Helpful Tip: In most cases, the slope will be expressed as an improper fraction in simplest form. Glance at the answer choices before you take the additional step of converting slope into a mixed number or decimal.
If you have time, double check your calculations against your rough sketch to make sure your answer makes sense. You’re done!




